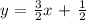If I am given a line with the points (1,2) and (-1,-1) how would I find out what the slope-intercept form is?

Question

asked Feb 1, 2023 69.3k views

1 Answer

Jon Goodwin · Answer 1 · 2023-02-06T21:41:39+0000

Solution:

The slope-intercept form of a line with slope m and y-intercept b is given by the following formula:

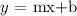
$y\text{ = mx+b}$

On the other hand, the slope m is given by the following equation:

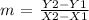
$m\text{ = }(Y2-Y1)/(X2-X1)$

where (X1,Y1) and (X2,Y2) are points on the line. In this case, we can take the points:

(X1,Y1) = (1,2)

(X2,Y2) = (-1,-1)

replacing this data into the slope equation, we get:

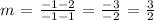
$m\text{ = }(-1-2)/(-1-1)\text{ = }(-3)/(-2)\text{ = }(3)/(2)$

thus, the slope of the line would be:

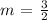
$m\text{ = }(3)/(2)$

now, replacing this into the slope-intercept form of the line we get:

EQUATION 1

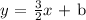
$y\text{ = }(3)/(2)x\text{ + b}$

We only need to find the y-intercept b. For that, take any point on the line, for example (x,y) = (1,2), and replace it into the previous equation:

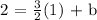
$2\text{ = }(3)/(2)(1)\text{ + b}$

this is equivalent to:

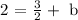
$2\text{ = }(3)/(2)+\text{ b}$

solving for b, we get:

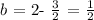
$b\text{ = 2- }(3)/(2)\text{ = }(1)/(2)$

that is:

$b\text{ = }(1)/(2)$

finally, replacing this into the EQUATION 1, we get:

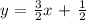
$y\text{ = }(3)/(2)x\text{ + }(1)/(2)$

then, the slope-intercept form of a line with the points (1,2) and (-1,-1) would be:

$y\text{ = }(3)/(2)x\text{ + }(1)/(2)$