Question

Solve the system using the substitution method-7 x - 2 y = -13 x - 2 y = 11

Answer 1

The solution is:

• x = 3

,

• y = -4

Step-by-step explanation

The substitution metod consists in clearing one variable from one of the equations, replacing the expression in the other equation and solving that new equation for the other variable.

Clearing x from the second equation:

`\begin{gathered} x-2y=11 \\ x=2y+11 \end{gathered}`

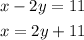
Replace x by this expression in the first equation:

`\begin{gathered} -7x-2y=-13 \\ -7(2y+11)-2y=-13 \end{gathered}`

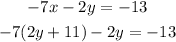
Solving for y:

`\begin{gathered} -14y-77-2y=-13 \\ -16y-77=-13 \\ -16y=77-13 \\ -16y=64 \\ y=(64)/(-16) \\ y=-4 \end{gathered}`

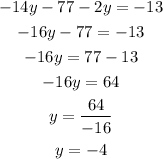
Now, we have to replace y = -4 in the first equation where we cleared x before:

`\begin{gathered} x=2y+11 \\ x=2(-4)+11 \\ x=-8+11 \\ x=3 \end{gathered}`

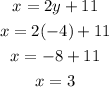
The solution is x = 3 and y = -4