Question

6. a) Calculate the areas of the three squares. b) Is the triangle a right triangle? Explain. 3 cm 4 cm 2 cm

Answer 1

The total area of the squares = 29 square cm

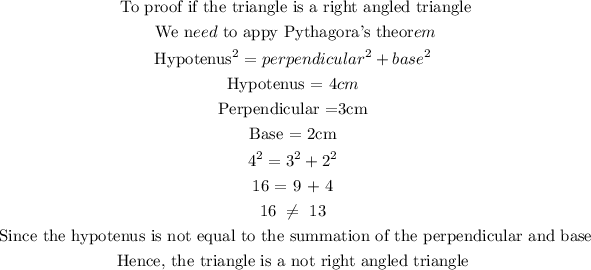
The triangle is not a right-angled triangle

Explanation:

We are given three squares

The first square has a length of 4cm

The second square has a length of 3cm

The third square has a length of 2cm

Part A

Total area = Area of square 1 + Area of square 2 + Area of square 3

Area of a square = l^2

Where l is the length of the square

Area of a square 1 = 4^2

Area of a square = 16 square cm

Area of square 2 = 3^2

Area of square 2 = 9 square cm

Area of square 3 = 2^2

Area of square 3 = 4 square cm

Total area of the square = 16 + 9 + 4

The total area of the square = 29 square cm

Hence, the total square of the figure is 29 square cm

Part B

`\begin{gathered} \text{ To proof if the triangle is a right angled triangle} \\ \text{ We n}eed\text{ to appy Pythagora's theor}em \\ \text{Hypotenus}^2=perpendicular^2+base^2 \\ \text{Hypotenus = 4}cm \\ \text{Perpendicular =3cm} \\ \text{Base = 2cm} \\ 4^2=3^2+2^2 \\ 16\text{ = 9 + 4} \\ 16\text{ }\\e\text{ 13} \\ \text{ Since the hypotenus is not equal to the summation of the perpendicular and base} \\ \text{Hence, the triangle is a not right angled triangle} \end{gathered}`