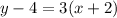Since a single line passes through two points, then you can first obtain the slope of the line using the formula
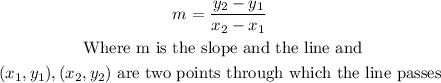
And then use the point-slope formula to find the equation of the line, that is

So, in this case, you have

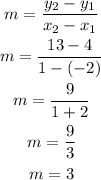
Now, using the point-slope formula
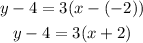
Therefore, the correct answer is A.