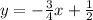Question

Find the equation line that passes through the points (-6,5)and (-2,2)

Answer 1

`y=-(3)/(4)x+(1)/(2)`

Step-by-step explanation:

A general equation for a line in the slope-intercept form is y = mx + b.

Given the points (-6, 5) and (-2, 2), we can substitute the first point in the equation:

`\begin{gathered} 5=-6m+b \\ 5+6m=b \\ b=5+6m \end{gathered}`

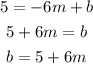
Now, we can substitute the second point in the equation and use the relation for "b" found above:

`\begin{gathered} y=mx+(5+6m) \\ 2=-2m+5+6m \\ 2-5=4m \\ -3=4m \\ m=-(3)/(4) \end{gathered}`

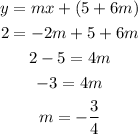
Since b = 5 + 6m, we can now find b:

`\begin{gathered} b=5+6\cdot(-(3)/(4)) \\ b=5-(18)/(4) \\ b=(4\cdot5-18)/(4)=(20-18)/(4) \\ b=(2)/(4)=(1)/(2) \end{gathered}`

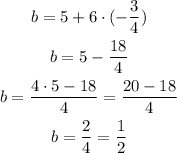
Thus, the equation of the line is:

`y=-(3)/(4)x+(1)/(2)`