Question

two tugboats are towing a ship. Each exerts a force of 6 tons, and the angle between the towropes is 60°. what is the resultant force on the ship?

Answer 1

10.39tons

Explanations:

The force exerted on the two tugboats F1 = F2 = 6tons

According to the cosine rule;

`R^2=F_1^2+F_2^2+2F_1F_2cos\theta`

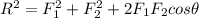
where

R is the resultant force

theta is the angle between the two ropes

Substitute the given parameters

`\begin{gathered} R^2=6^2+6^2+2(6)(6)cos60^0 \\ R^2=72+72cos60^0 \\ R^2=72+72(0.5) \\ R^2=72+36 \\ R^2=108 \\ R=√(108) \\ R=10.39tons \end{gathered}`

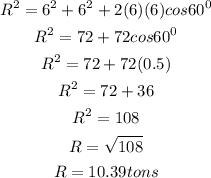
Therefore the resultant force on the ship is 10.39tons