Question

Julia drew a candy, recorded the color, and then replaced it before drawing again. She repeated this 12 times and only drew a red one time. Explain why there is a difference between the theoretical and experimental probabilities. What could Julia do to lessen the difference?

Answer 1

There are two terms to be understood i.e. experimental probability, and experimental probability.

Experimental probability is one which is calculated based on the real experiment results. While a theoritical probability is based upon logic and resoning.

In the given problem, it is given that Julia could draw a red candy only 1 out of 12 trials. So according to the concept of experimental probability, the probability of getting a red candy is 1/12.

Note that this is based on the real time experiment and results, so the probability used here is experimental probability,

`\begin{gathered} P(\text{ drawing a red candy)=}\frac{\text{ no. of times red candy is drawn}}{\text{ total no. of draws (or trials) made}} \\ P(\text{ drawing a red candy)=}\frac{\text{1}}{\text{ 1}2} \end{gathered}`

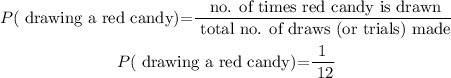
For the theoritical probability, we need to know how many red candies and total candies are there.

`P(\text{drawing a red candy)=}\frac{\text{ no. of red candies}}{\text{ total no. of candies}}`

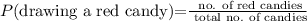
In the given problem, the details about total candies and red candies are not given, so we cannot calculate the theoritical probability.

Note that we don't have to actually perform the experiment to determine the theoritical probability. It is based on some logic and reasoning.

More the number of red candies in the lot, more will be the probability of drawing a red candy.

Note that the results from experimental probability approaches close to the results from theoritical probability, as the number of trials increase. At infinity, both the results will coincide.

So Julia could have increased the number of trials to lessen the difference.