B(3,-1)
Step-by-step explanation

Step 1
Point 1
(-2,0)
replace
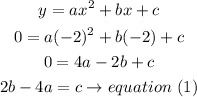
Point 2
(8,0)
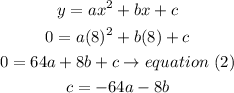
c) c= c, so
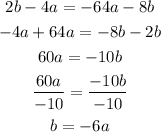
Step 2
when the function is in the form

the vertex is given by
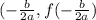
so,replace
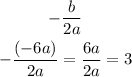
therefore, the component of the vertex is 3
let's check the options
A. (3,0)
B. (3,-1)
we can see that y component of option A is zero , it means (3,0 ) is a zero of the function, but we had already the zeros, therefore, we can discard this options,
in other words,
the answer is
B(3,-1)
I hope this helps you