Question

Sodium azide, NaN3, decomposes according to the following equation and produces N2 gas that inflates the SRS airbag when a car is involved in a serious collision. 2NaN3(s) 2Na(s) + 3N2(g) How many grams of sodium azide (molar mass=65.02 g/mol) is needed to inflate a 45.0-L airbag at 25C and a pressure of 809 torr? (Assume that the decomposition produces 100% yield.)

Answer 1

INFORMATION:

We know that:

- Sodium azide, NaN3, decomposes according to the following equation 2NaN3(s) → 2Na(s) + 3N2(g)

- We have an 45.0L airbag at 25°C and a pressure of 809 torr

And we must find how many grams of sodium azide are needed to inflate the airbag

STEP BY STEP EXPLANATION:

To find it, we need to use the Ideal Gas Law formula PV = nRT

Where,

P = pressure

V = volume

n = number of moles

T = temperature

R = gas constant

Is given that;

P = 809 torr

V = 45.0L

n = we need to calculate it

T = 25°C, converting to K, 25 °C + 273.15 = 298.15 K

R = 62.36 (L*torr)/(mol*K)

Now, replacing in the formula,

`809\text{ torr}\cdot45\text{ L}=n\cdot62.36(L\cdot torr)/(mol\cdot K)\cdot298.15K`

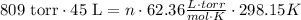
Now, solving for n

`n=(809torr\cdot45L)/(62.36(L\cdot torr)/(mol\cdot K)\cdot298.15K)=1.9580mol`

Now, using that molar mass of NaN3 = 65.02 g/mol, we can calculate the grams

`1.9580mol\cdot65.02(g)/(mol)=127.3092g`

Then, 127.3092 g are needed to inflate the airbag.

ANSWER:

127.3092 grams are needed to inflate the airbag.