We have to calculate the flow rate of the solution (in ml/hr) and the flow rate of the dextrous (in mg/hr).
We can find the flow rate of the solution as the ratio between the total solution given in 7 hours (1.358 L) and the period of time (7 hours):

As the solution is 15 mg per 100 mL of solution, we can multiply the flow rate of the solution by this proportion in order to calculate the flow rate of dextrous:
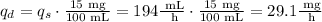
If each mL contains 15 drops, we can calculate the flowrate in gtt/mL multiplying the proportion of drops to mL by the flow rate of the solution:
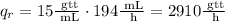
To calculate the amount of dextrous delivered in 7 hours we can multiply the flow rate by the period of time:
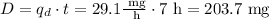
Answer:
Flow rate of the solution: 194 mL/h
Flow rate of dextrous: 29.1 mg/h
Flow rate in drops: 2910 gtt/h
Amount of dextrous in 7 hours: 203.7 mg