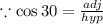In the given figure
There is a right triangle whose legs are 11, x, and its hypotenuse is y
The side opposite to the angle of measure 30 degrees is 11
The side adjacent to the angle of measure 30 degrees is x
Let us use the trigonometry ratios to find x and y
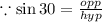
The opposite is 11 and the hypotenuse is y
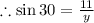
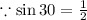
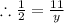
By using cross multiplication