Question

write the equation of a line perpendicular to the line that passes through the given point.y = -x + 20(-4,2)

Answer 1

The equation of a line can be written in the slope-intercept form as follows:

y=mx+b

where m is the slope, and b is the y-intercept

We are provided a line:

y=-x+20

Comparing with the general form, we have:

slope m=-1

intercept b=20

The line we are required to find must be perpendicular to the line above.

Two lines with slopes m1 and m2 are perpendicular if:

m1*m2=-1

That equation will give us the slope of our line. We only need to solve for m2 because we already have m1=-1

Solving for m2:

m2=-1/m1=-1/(-1)=1

This means the new line has a slope of 1.

The new line can be easily expressed by using the slope-point form of the line:

y=m(x-xo)+yo

Here, xo=-4, yo=2, thus

`y=\mleft(1\mright)\mleft(x-\mleft(-4\mright)\mright)+2`

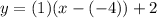
In plain text:

y=(1) [ x - (-4) ] +2

Simplifying:

y=x+4+2=x+6

The equation of the required line is

y=x+6