Given:
Find: which theorem verify that given two triangles are similar.
Step-by-step explanation:
by AA creteria, we have
angle A is equal to angle D i.e; 63 degree
angle C is equal to angle F i.e; 90 degree
In triangle ABC,
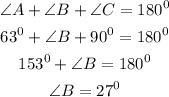
Similarly ,
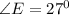
hence the given two triangles are similar by AA property.
Final answer: AA property is required answer.