Answer:
34 ft/s
Step-by-step explanation:
The average rate of change in height during the first second can be calculated as

Because the time goes from 0 to 1 second.
Using the given equation for h(t), we can calculate h(1) and h(0) as follows
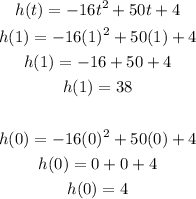
Then, the average rate of change in height is
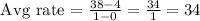
Therefore, the average velocity during the first second is 34 ft/s