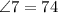Angles of the first line are the same of the second line because the lines are parallel and intersected by the same line
then
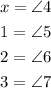
then
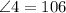
Now the opposite angles by the vertex like X and angle2 have the same value
then
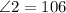
and
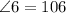
the adjacent angle of each angle make a straight angle then the sum is 180
now if we sum x and angle 1 we have 180
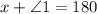
we replace the value of x and solve for angle 1

opposite angle by the vertex of angle1 is angle3, then

and angle3 and 7 are the same