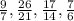Question

List the improper fractions in order from greatest to least:9/7, 7/6, 17/14, 26/21

Answer 1

`(9)/(7),(26)/(21),(17)/(14),(7)/(6)`

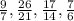
Explanation:

Given the improper fractions:

`(9)/(7),(7)/(6),(17)/(14),(26)/(21)`

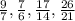
In order to arrange the fractions, first, find the lowest common multiple of the denominators.

• LCM of 7, 6, 14, and 21 = 42

Next, rewrite each fraction as an equivalent fraction using the LCM as the new denominator.

`\begin{gathered} (9)/(7)=(9*6)/(7*6)=(54)/(42) \\ (7)/(6)=(7*7)/(6*7)=(49)/(42) \\ (17)/(14)=(17*3)/(14*3)=(51)/(42) \\ (26)/(21)=(26*2)/(21*2)=(52)/(42) \end{gathered}`

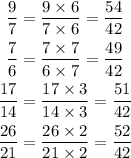
Since all the denominators are the same, order the numerators from the greatest to the least:

`\begin{gathered} (9)/(7)=(9*6)/(7*6)=(54)/(42) \\ (26)/(21)=(26*2)/(21*2)=(52)/(42) \\ (17)/(14)=(17*3)/(14*3)=(51)/(42) \\ (7)/(6)=(7*7)/(6*7)=(49)/(42) \end{gathered}`

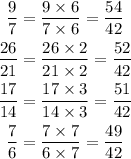
Thus, the fractions ordered from greatest to least is:

`(9)/(7),(26)/(21),(17)/(14),(7)/(6)`