Question

The rent in an urban neighborhood is normally distributed. The mean is $650 per month with a standarddeviation of $50. Estimate the percent of apartment residents who pay from $600 to $750 per month.Show Your Work

Answer 1

The z-score, also referred to as standard score, z-value, and normal score, among other things, is a dimensionless quantity that is used to indicate the signed, fractional, number of standard deviations by which an event is above the mean value being measured. Values above the mean have positive z-scores, while values below the mean have negative z-scores.

Mean is $650

Standard deviation = $50

`\begin{gathered} \mu=650 \\ \sigma=50 \end{gathered}`
`Z=(x-\mu)/(\sigma)=(600-650)/(50)=-(50)/(50)=-1`
`Z=(x-\mu)/(\sigma)=(750-650)/(50)=(100)/(50)=2`

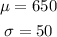
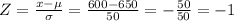
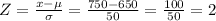
[tex]P(-1

Therefore the estimated percent of apartment residents = 81.86%