The average of the scores = sum/number
The scores are 88, 89, 91, 95
Their sum = 88 + 89 + 91 + 95 = 363
Let the score of the 5th quiz is x
Then the sum of the 5 quizzes = x + 363
Then the average of the 5 quizzes is
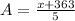
We need an average of at least 90, then
At least means greater than or equal

Substitute A by its value above
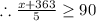
To solve it, multiply both sides by 5 to cancel the denominator on the left side
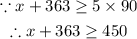
Subtract 363 from both sides to find x
