Question

In one day, a salesman plans to visit 6 out of 11 companies that are in the neighborhood.How many ways can he pick the visits?(He visits one at a time and makes a list to start)

Answer 1

From the question, we can deduce the following:

Number of companies in the neighbourhood = 11

Number of companies he plans to visit = 6

Let's determine the number of ways he can the the company he visits.

To determine the number of ways, apply the permutation formula:

`_nP_r=(n!)/(n-r!)`

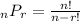
Where:

n = 11

r = 6

Thus, we have:

`\begin{gathered} _(11)P_6=(11!)/((11-6)!) \\ \\ _(11)P_6=(11!)/(5!) \\ \\ _(11)P_6=(11*10*9*8*7*6*5!)/(5!) \\ \\ _(11)P_6=11*10*9*8*7*6 \\ \\ _(11)P_6=332640 \end{gathered}`

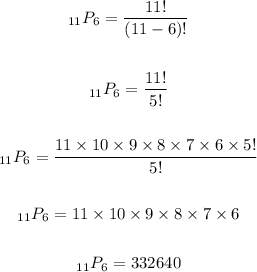
Therefore there are 332640 ways he can pick the visits.

ANSWER:

332640 ways