Question

A lake was stocked with 380 trout. Each year, the population decreases by 20. The population of trout in the lake after x years is represented by the function f(x)=380-20x.

Answer 1

x-intercept = -19

y-intercept = 380

Given

`f(x)=380-20x`

Part A

To find the x-intercept, we set y=0

The x intercept is the point where the line crosses the x axis. At this point y = 0

`\begin{gathered} y=380-20x \\ \text{when y=0 what is x } \\ 0=380-20x \\ 380=-20x \\ \text{rewrite} \\ -20x=380 \\ \text{divide both sides by -20} \\ -(20x)/(20)=(380)/(-20) \\ \\ x=-19 \end{gathered}`

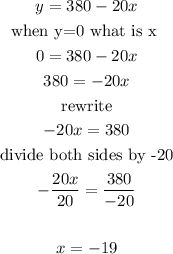
Part B

To find the y-intercept, we set x=0

The y- intercept is the point where the line crosses the x-axis.

At this point x= 0

`\begin{gathered} y=380-20x \\ x=0 \\ y=380-20(0) \\ y=380-0 \\ y=380 \end{gathered}`

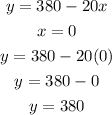
Part C

Graph of f(x)=380-20x