Answer:
(A)Cylinder 2 and the sphere
(B)4.8
Step-by-step explanation:

Cylinder 1
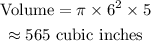
Cylinder 2

Cone 1
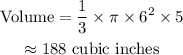
Cone 2
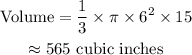
Sphere

Therefore: Cylinder 2 and the sphere have a volume greater than 600 cubic inches .
Part B
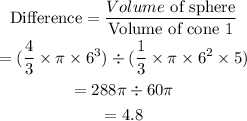
The volume of the sphere is 4.8 times greater than the volume of cone 1.