Question

What is the sum of the first 16 terms of the arithmetic sequence 11/5,8/5,1,2/5,…?A -59.2B -41.6c -36.8D -20.8

Answer 1

Given the sequence:

`(11)/(5),(8)/(5),1,(2)/(5),...`

We will check the type of the sequence arithmetic or geometric

`\begin{gathered} (8)/(5)-(11)/(5)=-(3)/(5) \\ \\ 1-(8)/(5)=-(3)/(5) \\ \\ (2)/(5)-1=-(3)/(5) \end{gathered}`

As shown there is a common difference = -3/5

So, it will be an arithmetic sequence.

We will find the sum of the first 16 terms using the following formula:

`S=(n)/(2)(2a+(n-1)d)`

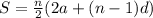
Substitute n = 16, d = -3/5, and a = 11/5

`S=(16)/(2)(2*(11)/(5)+(16-1)(-(3)/(5)))=-36(4)/(5)=-36.8`

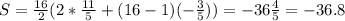
So, the answer will be option c) -36.8