Question

Perform the indicated operation by removing the parentheses and combining the like terms.(7x4 + 2x3) - (-9x4 + 5x3)

Answer 1

We are given the following expression:

`\mleft(7x^4+2x^3\mright)-(-9x^4+5x^3)`

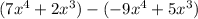
To remove the parenthesis we will apply the distributive law on the right parenthesis:

`(7x^4+2x^3)-(-9x^4+5x^3)=7x^4+2x^3+9x^4-5x^3`

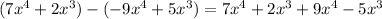
Now, we associate like terms. This means the terms that have the same variable and that are elevated to the same exponent.

`7x^4+2x^3+9x^4-5x^3=(7x^4+9x^4)+(2x^3-5x^3)`

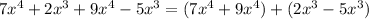
Now, we add like terms:

`(7x^4+9x^4)+(2x^3-5x^3)=16x^4-3x^3`

Since we can't simplify any further this is the final answer.