Question

Suppose the $2000 is loaned at a rate of 16% compounded semiannually. Assuming that no payments are made, find the amount owed after 3 years. Round your answer to the nearest cent.

Answer 1

`\$3,173.75`

Step-by-step explanation

We want to find the amount owed after 3 years.

To find this, we apply the formula for compound interest:

`A=P(1+(r)/(n))^(nt)`

where P = principal = $2000

r = rate = 16% = 0.16

n = number of times it is compounded per year = 2

t = number of years = 3

Therefore, we have that:

`\begin{gathered} A=2000(1+(0.16)/(2))^((2\cdot3))_{} \\ A=2000(1+0.08)^6 \\ A=2000(1.08)^6 \\ A=\$3,173.75 \end{gathered}`

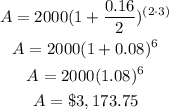
That is the amount owed after 3 years (to the nearest cent)