Question

in a square garden plot (with an area of 400 sq ft)deer fencing priced at $1.50 per foot is to be installed around the plot. if sales tax is 7% how much will fencing price cost? (do not round)

Answer 1

We have a square garden of 400 square foot.

The area of a square is:

`A=x^2`

where x: side length.

In this case:

`\begin{gathered} A=400=x^2 \\ x=\sqrt[]{400}=20\text{ ft} \end{gathered}`

The perimeter of the square is the sum of the lengths of the sides of the square. As they are all equal, we can write:

`P=4x=4\cdot20=80\text{ ft}`

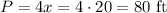
The fencing is priced at $1.50 per foot. If we add the 7% sales tax to this price we get:

`C=1.50\cdot(1+0.07)=1.50\cdot1.07=1.605\text{ \$/ft}`

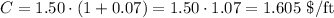
The fencing will be installed in all the perimeter (80 ft).

We can calculate the total cost by multiplying the sales price ($1.605 per foot) and the perimeter (80 ft):

`F=C\cdot P=1.605\frac{\text{\$}}{\text{ft}}\cdot80\text{ ft}=\$128.40`

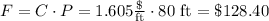
Answer: the fencing will cost a total of $128.40