Solution:
Given the complex number:
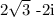
Step 1: Express the complex number in polar form.
In polar form, we have
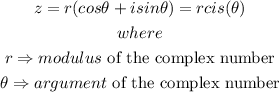
Step 2: Evaluate the modulus of the complex number,
The modulus of the complex number is expressed as

Step 3: Evaluate the argument of the complex number.
The argument of the complex number is expressed as
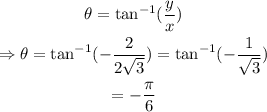
Thus, in polar form, the complex number becomes
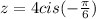
To evaluate the fourth root, we use the De Moivres's theorem.
According, to the DeMoivres's threorem,

In this case,
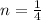
Thus,
![undefined]()