Question

For the parallelogram, if m22 - 4x -22 and m<4 - 3x - 6, find m23 The diagram is not toscale.

Answer 1

`m\angle2+m\angle3=180^0(Adjacent\text{ sides of a parallelogram are supplementary)}`
`m\angle2=m\angle4\text{ (opposite angles of a parrallelogram are equal)}`

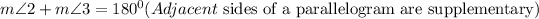
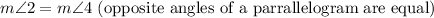
We going to use the second condition to obtain the value of x first, then go back to the first condition to obtain the required measure of angle 3.

Thus, we have:

`\begin{gathered} m\angle2=m\angle4 \\ 4x-22=3x-6 \\ 4x-3x=-6+22 \\ x=16^0 \end{gathered}`

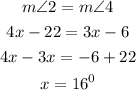
Having gotten the value of x, then we can now find the required measure of angle 3.

Thus, we have:

`\begin{gathered} m\angle2+m\angle3=180^0^{} \\ 4x-22+m\angle3=180 \\ m\angle3=180-4x+22 \\ x\text{ has b}een\text{ to be 16} \\ m\angle3=180-4(16)+22 \\ m\angle3=180-64+22 \\ m\angle3=138^0 \end{gathered}`

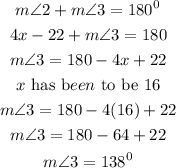
Hence, the correct option is option A