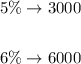Given:
Total invested amount = $9000
One account = 5%
Another account = 6%
Find-:
Invested amount of each equation
Explanation-:
Let in 5% account invested amount is x
then amount in 6% account is (9000-x)
Then,
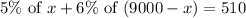
Then the value of "x" is:

The "x" is:
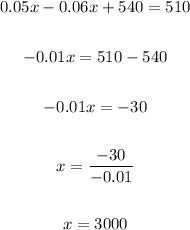
The value of x is 3000 the mean amount in 5% invested amount is 3000.
6% invested amount is:
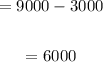
So each account invested amount is: