In the similar triangles
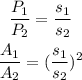
Since the ratio between the perimeters of 2 similar triangles, ABC and A1B1C1 is 4/5
By using the first rule above, the ratio between their sides is 4/5
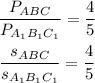
Since the sum of their area is 410 cm^2, then
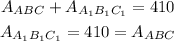
By using the 2nd rule above
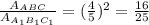
Substitute Area of triangle A1B1C1 by 410 - ABC
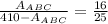
By using the cross-multiplication
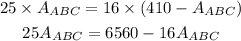
Add 16A (ABC) to both sides
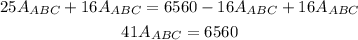
Divide both sides by 41
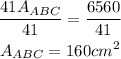
Subtract it from 410 to find the area of triangle A1B1C1
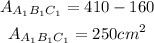
The areas of the 2 triangles are 160 cm^2 and 250 cm^2