Question

If the length of a rectangular parking lot is 10 meters less than twice its width, and the perimeter

is 400 meters, find the dimensions of the parking lot?

Answer 1

The final result is Width = 70 and Length = 130

Explanation:

First, set up an algebraic equation. Lets call length L and width W and perimiter P.

The equation for the perimiter of a rectangle is 400 = 2L + 2W

Since we have clues about the dimentions, we can edit the equation.

Length is 10 meters less than twice the width.

L = 2W - 10

By substitution, you can put L = 2W - 10 in for the L in 400 = 2L + 2W.

This would give you 400 = 2(2W -10) + 2W

Now you want to distribute the 2. This gives you 400 = 4W - 20 + 2W.

After that, add up the Ws 400 = 6W - 20.

Move the 20 over to the other side.

420 = 6W

Divide both sides by 6

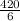`(420)/(6)` = W

W = 70

Now put 70 in for W in the original equation and solve for L.

400 = 2(70) + 2L

Simplify

400 = 140 + 2L

260 = 2L

130 = L

The final result is Width = 70 and Length = 130