Question

(20pts, 5pts each) The median of AMND from vertex N of the

isosceles trapezoid MNFD is 5cm. The diagonal ND is
perpendicular to the leg MN. The shorter base NF = 6cm and the
32cm².
A
MNFD
=
Find: MD, the altitude of the trapezoid h, the length of the
legs of the trapezoid and the ratio between the areas of AMND and
ANFD.

Answer 1

• MD = 10 cm
• h = 4 cm
• MN = FD = 2√5 cm ≈ 4.47 cm
• area ratio = 5 : 3

Explanation:

Given isosceles trapezoid MNFD with short base NF = 6 cm, diagonal ND perpendicular to leg MN, and triangle MND median NX = 5 cm, you want ...

• length MD
• altitude h of the trapezoid
• lengths of MN and FD
• ratio of areas of ∆MND and ∆NFD

MD

MD is the hypotenuse of right triangle MND. The median from the right angle of a right triangle is half the length of the hypotenuse, so ...

MD = 2·NX = 2·(5 cm)

MD = 10 cm

Altitude

In the attached diagram, we see that right triangle XYN has side XY = 3 (half of NF), and hypotenuse NX = 5 (given). These are two sides of a 3-4-5 right triangle, so the remaining side, altitude h, is 4.

h = 4 cm

Side lengths

The attache diagram shows congruent side lengths MN and FD are the hypotenuse of a right triangle with sides YM = 2 and YN = 4. The Pythagorean theorem tells us the length of side MN is ...

MN² = MY² +NY²

MN² = 2² +4² = 4 +16 = 20

MN = √20

MN = FD = 2√5 ≈ 4.47

Area ratio

Triangle MND has a base length MD = 10 and a height h = 4. Triangle NFD has a base length NF = 6 and a height h = 4. The ratio of the areas of these two triangles is ...

`(A_(MND))/(A_(NFD))=((1)/(2)\cdot10\cdot4)/((1)/(2)\cdot6\cdot4)=(10)/(6)=\boxed{(5)/(3)}`

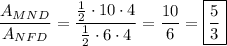
The ratio between the areas is 5 : 3.

__

Additional comment

We are also given the area of the trapezoid as 32 cm². That can be used to find the longer base and/or the altitude, or to check the answers we have for those values. It is redundant information.

The midpoint X of MD is the center of the circumscribing circle of the trapezoid and right triangles MND and MFD. The midpoint of the hypotenuse of a right triangle is always the circumcenter, so knowing the length NX tells us the radius of that circle, hence MX and XD.