Answer:
For the CFL bulb, the cost at 0 hours will be
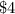 , and
, and
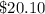 at
at
 hours.
hours.
For the LED bulb, the cost at 0 hours will be
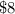 , and
, and
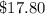 at
at
 hours.
hours.
Explanation:
Step 1: Calculating the cost for 0 hours
At
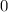 hours, the bulbs aren't at use, and so none of them are utilizing any energy.
hours, the bulbs aren't at use, and so none of them are utilizing any energy.
The lack of the use of energy, means no money has to be paid for the energy.
Thus, at
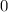 hours, both bulbs have a cost that is equal to their subsequent prices:
hours, both bulbs have a cost that is equal to their subsequent prices:
CFL bulb:
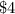
LED bulb:
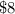
Step 2: Calculating the cost for 10,000 hours
For the CFL bulb,
 kWh is used within an hour.
kWh is used within an hour.
So within
 hours, the amount of energy will be:
hours, the amount of energy will be:
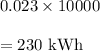
 cents is charged for each kWh, so for
cents is charged for each kWh, so for
 kWh, the cost will be:
kWh, the cost will be:
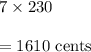
 dollar equals to
dollar equals to
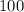 cents, so the cost found above in dollars will be:
cents, so the cost found above in dollars will be:

So, the total cost for the CFL bulb, along with its original price will be:
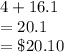
Similarly, for the LED bulb,
 kWh is used within an hour.
kWh is used within an hour.
So, within
 hours, the amount of energy will be:
hours, the amount of energy will be:
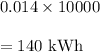
 cents is charged for each kWh, so for
cents is charged for each kWh, so for
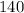 kWh, the cost will be:
kWh, the cost will be:
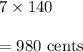
The cost found in dollars will be:

So, the total cost for the LED bulb, along with its original price will be:
