Question

Solve for x.3x + 2X + 28Since the angles are congruent, we can set theirequations equal to each other.3x + 2 = x + 282x + 2 = 282x = 26x = [?]=Hint: Divide each side of the equation by 2 for the final value of x.

Answer 1

To answer this question, you have that both equations can be equated since they represent the same angle since both lines are parallel. Then, we can write:

`3x+2=x+28`

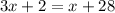
We need to solve for x, first, by subtracting x from both sides of the equation, and then subtracting 2 from both sides of the equation:

`3x-x+2=x-x+28\Rightarrow2x+2=28`

`2x+2-2=28-2\Rightarrow2x=26`

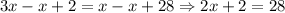
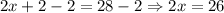
Finally, to find the value of x, we need to divide both sides by 2 as follows:

`(2x)/(2)=(26)/(2)\Rightarrow x=13`

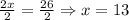
Therefore, the value for x = 13.