Find C Find A and find ab=21 c=26 B=34 degrees

Question

asked Dec 16, 2023 62.9k views

1 Answer

Laurine · Answer 1 · 2023-12-21T16:03:42+0000

The Law of Sines states that:

$(\sin A)/(a)=(\sin B)/(b)=(\sin C)/(c)$

Procedure

0. Using the Law of Sines to ,find C

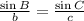
$(\sin B)/(b)=(\sin C)/(c)$

As we have the values of all variables but C, we isolate for it:

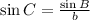
$\sin C=(\sin B)/(b)$

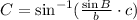
$C=\sin ^(-1)((\sin B)/(b)\cdot c)$

Replacing the values:

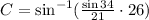
$C=\sin ^(-1)((\sin34)/(21)\cdot26)$

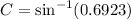
$C=\sin ^(-1)(0.6923)$

2. Calculating A

Then, knowing that all the interior angles of a triangle add up to 180°...

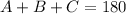
...we can isolate for A:

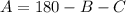
Replacing the values given for B and the value of C:

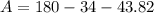
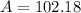
3. Calculating a

Now that we know A, we can find a using the Law of Sines:

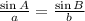
$(\sin A)/(a)=(\sin B)/(b)$

Isolating for a we get:

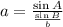
$a=(\sin A)/((\sin B)/(b))$

Replacing the values:

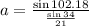
$a=(\sin 102.18)/((\sin 34)/(21))$

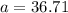
Answer:

• C = 43.82°

,

• A = 102.18°

,

• a = 36.71