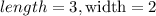SOLUTION
Given the question in the image, the following are the solution steps to answer the question
STEP 1: Write the given sides
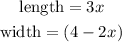
STEP 2: Calculate the area
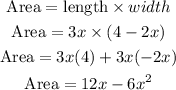
STEP 3: Calculate the maximum area of the rectangle
Set the differential to zero to get x
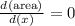
STEP 4: Find the first differential of the derived Area in step 2
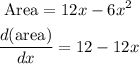
STEP 5: Set the differential to zero to get x
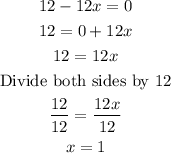
STEP 6: Substitute 1 for x in the area formula in Step 2
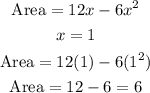
Hence, the maximum area of the reactangle is 6
STEP 7: Get the vertex
Using the formula for area in step 2

Hence, the vertex is (1,6)
STEP 8: Find the dimensions of the rectangle
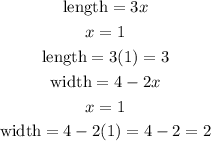
Hence, the dimensions of the rectangle are: