Question

Find the missing side of the triangle in the simplest radical form.

Answer 1

In a right angle triangle, the hypotenuse is always the side opposite the right angle

Hence, from the image given,

`\text{hypotenuse =x}`

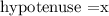
Apply pythagoras rule i.e the square of the hypotenuse side is equal to the sum of squares of the other two sides“

we have

`x^2=3^2+(2\sqrt[]{2})^2`

Simplify the expression above

`\begin{gathered} x^2=9+4(2) \\ x^2=9+8 \\ x^2=17 \end{gathered}`

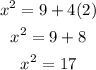
Take the square root of both sides

`\begin{gathered} \sqrt[]{x^2}=\sqrt[]{17} \\ \text{Then} \\ x=\sqrt[]{17}in \end{gathered}`

Hence

The missig side in the triangle is √17in

Answer: √17in