Question

explain how the graph, domain, and range change when you go from graphing the square root function to the cube root function.

Answer 1

Let us take

`√(x)`

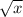
as an example.

The graph of the function will be shown below

Let us now get the domain and range of the function

The domain of the function is

`\:[0,\:\infty \:)`

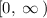
The range of the function is

`[0,\:\infty \:)`

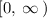
Let us now take the

`\sqrt[3]{x}`

as an example.

Let us graph the function

Let us now get the domain and range of the function

The domain of the function is,

`\:\left(-\infty \:,\:\infty \:\right)`

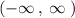
The range of the function is,

`\left(-\infty \:,\:\infty \:\right)`

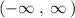
Note: The domain and the range of square-root of x starts from 0 to positive infinity while the domain and the range of cube-root of x starts from the negative infinity to positive infinity.