Question

Please help me with this it's on volume of composite figures/word problems

Answer 1

We separate the figure into two parts to be able to calculate each volume and at the end add them

triangular prism

Volume is area of the triangle by the length

`V=(b* h)/(2)* l`

where b is the base of the triangle, h the height of the triangle and l the the depth of the prism

replacing

`\begin{gathered} V=(40*10)/(2)*50 \\ \\ V=(400)/(2)*50 \\ \\ V=10000 \end{gathered}`

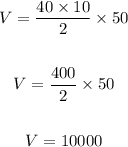
the volume is 10,000 cubic feet

Rectangular prism

Volume is the area of the rectangle by the length

`V=b* h* l`

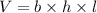
replacing

`\begin{gathered} V=40*15*50 \\ V=30000 \end{gathered}`

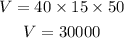
the volume of the rectangular prism is 30.000 cubic feet

Total Volume

we add the volume of both figures

`\begin{gathered} V=10000+30000 \\ V=40000 \end{gathered}`

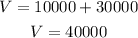
The total Volume is 40,000cubic feet