Question

Heights of men are normally distributed with a mean of 75 in and a standard deviation of 3.5 in.a) Find the height separating the bottom 90% from the top 10%.

Answer 1

In order to calculate the height separating the bottom 90% from the top 10%, first let's find the equivalent z-score for a probability of 90% in the z-table.

Looking at the z-table, the z-score for a probability of 90% is equal to approximately 1.28.

Then, to calculate the height, let's use the formula below for the z-score:

`\begin{gathered} z=(x-\mu)/(\sigma) \\ 1.28=(x-75)/(3.5) \\ x-75=1.28\cdot3.5 \\ x-75=4.48 \\ x=4.48+75 \\ x=79.48\text{ in} \end{gathered}`

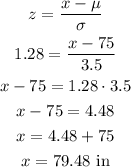
So the wanted height is equal to 79.48 inches.