Question

Which of the following sets of numbers could not represent the three sides of a right triangle? O {36, 84, 91} O {40, 75, 85} Submit Answer O {20, 21, 29} O {3,4,5)

Answer 1

Given:

The objective is to find which of the set of numbers does not represent the three sides of a right triangle.

The sides can be verified using Pythagoras theorem.

The formula of Pythagorean theorem is,

`\text{Hypotenuse}^2=Opposite^2+Adjacent^2`

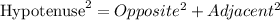
Consider the larger side as hypotenuse of the right triangle.

In option (A), the given point is (36,84,91).

`\begin{gathered} 91^2=36^2+84^2 \\ 8281=1296+7056 \\ 8281\\e8352 \end{gathered}`

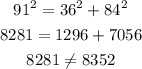
Since, in the above Pythagorean theorem, LHS is not equal to RHS.

Hence, option (A) could not represent the three sides of a right triangle.