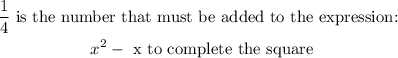Question

What number must be added to the expression below to complete thesquare?x²-x

Answer 1

Step 1:

In this question, we are given the following:

What number must be added to the expression below to complete the

square?

x²-x

Step 2:

Steps in completing the square method are as follows:

`\begin{gathered} \text{Given } \\ \text{x }^2\text{ - x} \\ We\text{ can s}ee\text{ that:} \\ \text{coefficient of x}^2\text{ = 1} \\ \text{Divide through by 1, we have that:} \\ x^2\text{ - x} \\ \text{Next:} \\ \text{coefficient of x = -1} \\ \text{Divide by 2, we have: }(-1)/(2) \\ Square\colon\text{(}(-1)/(2))^2\text{ =}(1)/(4) \end{gathered}`

Step 3:

From step 2, we can see that:

`\begin{gathered} (1)/(4)\text{ will be added to:} \\ x^2\text{ - x, } \\ \text{we have that:} \\ x^2-x\text{ + (}(1)/(4))^{}\text{ = ( x -}(1)/(2))^2 \end{gathered}`

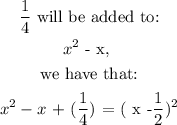
CONCLUSION:

`\begin{gathered} (1)/(4)\text{ is the number that must be added to the expression: } \\ x^2-\text{ x to complete the square} \end{gathered}`