Answer:
12. y = 3x + 5
Step-by-step explanation:
Two lines are parallel if they have the same slope.
In an equation of the form y = 3x + 1, the equation is the number beside the x, so the slope is 3. Then our line will have a slope of 3 and pass through the point (-1, 2).
The equation of a line with slope m that passes through the point (x1, y1) is

Replacing m = 3 and (x1, y1) = (-1, 2), we get:
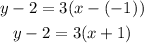
To write in slope-intercept form, we need to solve the equation for y, so

So, the answer for question 12 is y = 3x + 5