Question

The table below shows the cost C to company A, in dollars, of selling x cups of coffee per day from a cart . a. Assuming the function C is linear. What is the slope of the line corresponding to the graph of y = C(x)? The line of slope = ________

Answer 1

0.25

When x is 200, C is 90

Step-by-step explanation

The given function: y = C(x)

Using the following coordinates from the given table;

(5, 41.25) and (10, 42.5)

The slope of the line corresponding to the graph y = C(x) is calculated as shown below

`\begin{gathered} m=(\Delta y)/(\Delta x)=(y_2-y_1)/(x_2-x_1) \\ m=(42.5-41.25)/(10-5) \\ m=(1.25)/(5) \\ m=0.25 \\ \text{That is the slope is 0.25} \end{gathered}`

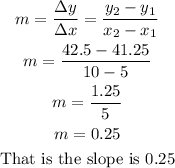
To get the value of C, when x is 200 in the chart, we shall calculate it as follows

`\begin{gathered} \text{Equation of a linear graph is:} \\ y=mx+c \\ \Rightarrow C=mx+c \\ \text{Where;} \\ m\text{ is the slope = 0.25} \\ x\text{ is the independent variable} \\ c\text{ is the C-intercept} \\ C\text{ is the dependent variable} \end{gathered}`

To get c, use (5, 41.25)

`\begin{gathered} 41.25=0.25(5)+c \\ 41.25=1.25+c \\ c=41.25-1.25 \\ c=40 \end{gathered}`

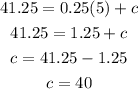
Therefore,

C = 0.25(200) + 40

C= 50 + 40

C = 90