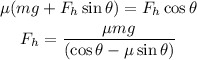Question

Find the magnitude Fh of the force required to slide the lawnmower over the ground at constant speed by pushing the handle

Answer 1

Given data

*The given mass of the lawnmower is m

*The given coefficient of friction is

`\mu`

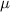
*The force exerted by the handle is

`F_h`

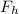
The free-body diagram is given below

The net force acting in the horizontal direction is given as

`\begin{gathered} f-F_h\cos \theta=0 \\ \mu N-F_h\cos \theta=0 \\ \mu N=F_h\cos \theta \end{gathered}`

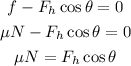
The net force acting in the vertical direction is given as

`mg+F_h\sin \theta=N`

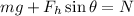
Now, Substitute the values in the horizontal direction of the forces equation as we get

`\begin{gathered} \mu(mg+F_h\sin \theta)=F_h\cos \theta \\ F_h=(\mu mg)/((\cos \theta-\mu\sin \theta)) \end{gathered}`