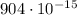We are told that we want the number 0.000 000 000 000 904 to be written in scientific notation. This happens mostly when a number has a big amount of zeros, so we want to avoid writing so many of them.
To write a number in scientif notation, we first identify the part of the number that does not have many consecutive zeros. In our case, that is 904.
Now, we want to use the following: Numbers that have an implicit decimal point /comma. For example, the number 10 is also written as 10.0, and we can move the decimal point to the right or the left by multiplying or dividing by 10.
Consider the number 2.3 for example.
If we multiply it by 10, we get
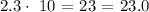
so the decimal point moved one space to the right. So if we multiply

so the comma moved 2 spaces to the right.
In general, mutliplying by 10^a where a is a positive number moves the decimal point a spaces to the right. If the number a is negative, then we are moving the decimal point to the left. For example
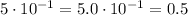
so we moved the decimal point 1 space to the left.
Going back to our case, we first write the non zero part of the number (904) and now we want to determine the number of spaces we want to move the decimal point. Since our number is 0.000 000 000 000 904 , we want to move the decimal point to the left. This means that the power of ten should be negative. Something of this form
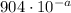
where a is the number of spaces we want to move the decimal point to the left.
Notice that there are 12 zeros to the left of 904, so we should at least move it 12 spaces, but since 904 = 904.0, we should also take into account the number of digits of the number 904. That is, we should move the decimal point a total of 12+3 spaces (namely 15).
Then, the final answer (but one of many possible answers) is