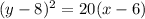Answer:
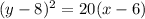
Step-by-step explanation:
Note that any point on the parabola is equidistant from the focus and directrix.
Distance from the point (x, y) to the directrix x = 1 is (x-1)
Also, distance from the point (x, y) to the focus (11,8) is expressed as;

Hence the required equation of the parabola in any form is expressed as;