2)
Given:
The diameter of larger circle, D=8.
Hence, in the given figure, the diameter of the smaller semicircle, D=8.
The diameter of smaller circle, d=4.
Hence, the diameter of the two smaller semicirlces, d=4.
The radius of the smaller semicircle is,
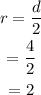
Now, the area of the smaller semicircle can be calculated as,
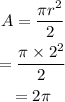
The radius of the larger semicircle is half its diameter. So, the radius of the larger semicircle is R=4.
Now, the area of the large semicircle is,
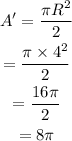
The two smaller semicircles are darkly shaded.
Hence, the area of the lightly shaded region can be found as,
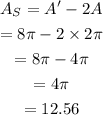
Therefore, the area of the lightly shaded region is 4π square units or 12.56 square units.
3)
Given:
The central angle is, θ=73 °.
From part (2), the radius of larger circle, R=4.
The area of the sector with radius R=4 and central angle, θ=73° is,
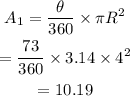
From part 2, the radius of the smaller circle is, r=2.
The area of the sector with radius r=2 and central angle, θ=73° is,
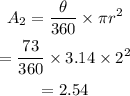
Now, the area of the lightly shaded region is,
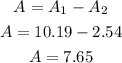
Therefore, the area of the lightly shaded region is 7.65 square units.