Answer:
Given that,
Gerald purchases a rectangular plot of land.
The length of the plot is 20 feet more than the width.
Let l and w be the length and width of the rectangle respectively in feet.
we get, l=w+20
The cost of the land was $12 per square foot. Gerald also had a fence put around the entire perimeter of the plot, at a cost of $8 per linear foot.
The total amount he spent on both the land and the fence was $10,560
we get,
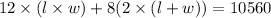
Substitute l, we get,
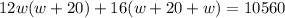
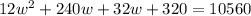
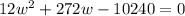
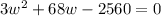

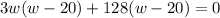
we get,
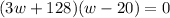
The possible width of the rectangle is 20 feet.
Length of the width is 40 feet.
The required dimension of the rectangle is ,
Length is 40 feet and width is 20 feet.