Question

can you help me with this math problem please the Fifth and tenth terms of an arithmetic sequence respectively are - 2 and 53 what is the seventh term of the sequence?

Answer 1

The fifth and tenth terms of an arithmetic sequence respectively are - 2 and 53 what is the seventh term of the sequence?​

Answer :

Step 1:

Using the nth term of an Arithmetic Progression,

`\begin{gathered} T_{n\text{ }}=\text{ a + ( n - 1 ) d, } \\ \text{where a = first term} \\ \text{ d = common difference} \\ T_{5\text{ }}=\text{ -2 , n = 5} \\ T_{\text{ 5 }}=\text{ a + ( 5 - 1 ) d = -2 } \\ a\text{ + 4 d = -2 ------ equ 1} \end{gathered}`

On the other hand,

`\begin{gathered} T_{n\text{ }}=\text{ a + ( n- 1 ) d} \\ n\text{ = 10} \\ T_(10)\text{ = a + ( 10 - 1 ) d = 53} \\ a\text{ + 9 d = 53 ------- equ 2} \end{gathered}`

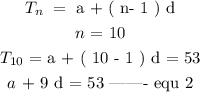
Step 2 :

We need to solve the two sets of linear equations, we have that: