Question

1) Find the total surface area of the following figure. Round your answer to thenearest whole number. .471 in2.432 in2.498 in2.456 in2

Answer 1

• Question 1

Let's write the formula you will use to find the volume of one cone.

To find the volume of a cone, use the formula below:

`V=(1)/(3)\pi r^2\sqrt[]{s^2-r^2}`

Where:

V is the volume

r is the radius of the cone

s is the slant height of the cone

• Question 2:

Let's use the formula above to find the volume of cone B from the given figure.

From cone B, we are given:

Diameter of cone B = 10 cm

Slant height of cone B, s = 10 cm

To find the radius of the cone, divide the diameter by 2:

`\text{Radius, r = }(diameter)/(2)=(10)/(2)=5\text{ cm}`

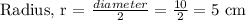
Thus, to find the volume, we have:

`V=(1)/(3)\pi\ast5^2\sqrt[]{10^2-5^2}`

Solving further, we have:

`\begin{gathered} V=(1)/(3)\pi\ast25^{}\sqrt[]{100-25} \\ \\ V=(1)/(3)\pi\ast25\sqrt[]{75}^{} \\ \end{gathered}`

Solving further:

`\begin{gathered} V=(1)/(3)\pi\ast25\sqrt[]{25\ast3}^{} \\ \\ V=(1)/(3)\pi\ast25\sqrt[]{5^2\ast3} \\ \\ V=(1)/(3)\pi\ast25\ast5\sqrt[]{3} \\ \\ V=(1)/(3)\pi\ast125\sqrt[]{3} \end{gathered}`
`\begin{gathered} V=\frac{\pi\ast125\sqrt[]{3}}{3} \\ \\ \text{ V = 226.72 cm}^3 \end{gathered}`

Therefore, the volume of cone B is 226.72 cubic centimeters